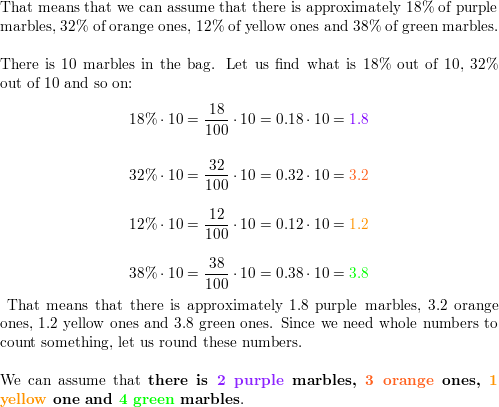First since we are dealing with the color orange and the rest according to the question we can say that we have only two sets of marbles.
A bag contains 5 red marbles 3 green marbles 2 purple marbles 2 orange marbles and 1 blue marble.
Two marbles are drawn without replacement.
In this bag with x 2 5 marbles total x 1 are red.
A bag contains 5 green marbles 8 red marbles 11 orange marbles 7 brown marbles and 12 blue marbles.
B find probabilities for p bb p br p rb p ww p at least one red p exactly one red 3.
What is the probability of choosing a red marble if a single choice is made from the bag.
A jar contains 4 black marbles and 3 red marbles.
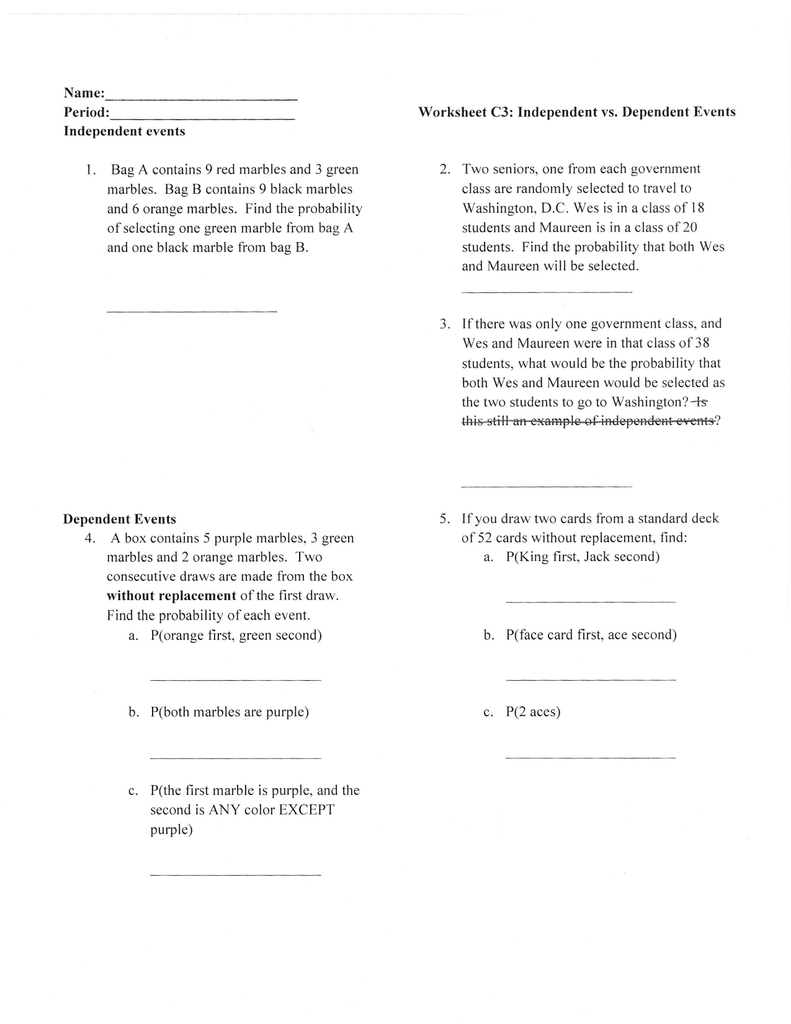
A box contains 5 purple marbles 3 green marbles and 2 orange marbles.
A draw the tree diagram for the experiment.
P 1st orange then green.
What is the probability of randomly selecting a blue marble then without replacing it randomly selecting a green marble.
A bag contains 3 red marbles 5 green marbles and 2 blue marbles.
Bag a contains 9 red marbles and 3 green marbles.
Algebra linear inequalities and absolute value theoretical and experimental probability.
A marble is randomly drawn and then replaced.
Report answers to 3 decimal places.
A marble is chosen at random from the jar and replaced.
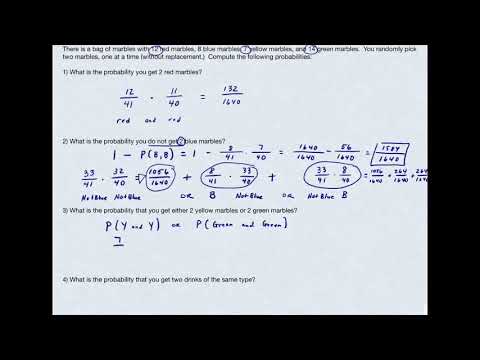
A bag contains 8 red marbles 5 blue marbles 8 yellow marbles and 6 green marbles.
A box contains 5 purple marbles 3 green marbles and 2 orange marbles.
The second set contains 5 blue and 4 red marbles making a total of 9.
A bag contains 5 blue marbles 4 red marbles and 3 orange marbles.
Find the probability that both are blue under each condition.
Two marbles are drawn without replacement from a jar containing 4 black and 6 white marbles.
The first set is the orange and that contains just 3 orange marbles.
5 red marbles 6 blue marbles 3 green marbles 4 black marbles 2 yellow marbles a marble will be drawn from the bag and replaced 100 times.
Draws are made without replacement.
Two marbles are drawn one at a time from the bag.
3 yellow marbles and 2 black marbles.
Is it 8 27.
After drawing out the first marble it is replaced in the bag before drawing the second marble.
A bag contains 3 red marbles 2 blue marbles and 5 green marbles.
A bag contains 5 red marbles and 4 pink marbles.
Draws are made without replacement.
The probability of drawing a red marble from the original bag is equal to that.
Also x 3 marbles have a scratch on them.